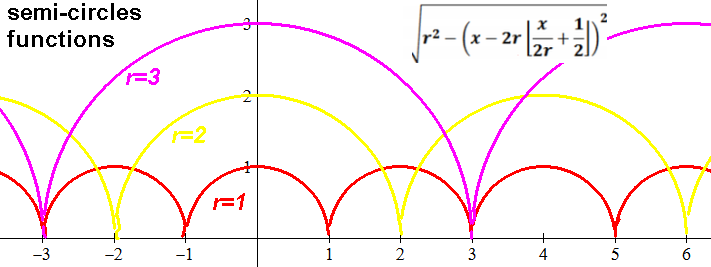

Periodic with period 2r.
Continuous everywhere but not differentiable at the cusps/kinks
(at which the tangent lineis vertical, thus slope if undefined).

Note the floor function in the definition.
Probably why these semicircle functions aren't
of the usual suspects in math classes,
as how are they differentiated?
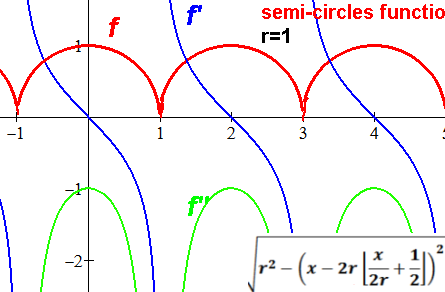
ƒ' was simulatd here by -x/sqrt(1-x^2) and shifted by multiples of 2
ƒ'' by -1/sqrt(1-x^2) - x^2/(1-x^2)^(3/2) and shifted by multiples of 2
In each period, i.e. each semicircle, i.e. from a cusp to the next, the function is increasing in the first half,
flattens at the midpoint, then is decreasing to the end.
In each period the slope of the tangent line at each point on
the function, indicated by ƒ', is decreasing from
positive infinity at the cusp at the start of the period to negative infinity
at the cusp at the end of the period.
Critical points:
The slope, i.e. the ƒ' value, is 0
at the midpoint of the semicircle, and it's a local maximum with value r.
The slope is undefined
at the cusps at the start and end of each period, and they are local minima
with value 0.
The second derivative ƒ'' is always negative, indicating the function is always
concave down and thus that it has no inflection points.
Flipped/negated semicircles and first and second derivatives:



| [0,π] | semicircle
r=π/2 | sin |
|---|---|---|
| arc length | π2/2≈ 4.935 | ≈ 3.82 |
| area under curve | π3/8≈ 3.876 | 2 |
| area of surface of revolution | sphere of radius π/2
π3≈ 31.006 | ≈ 14.42 |
| volume of revolution | sphere of radius π/2
π4/6≈ 16.235 | ≈ 4.935 |